Table of Contents
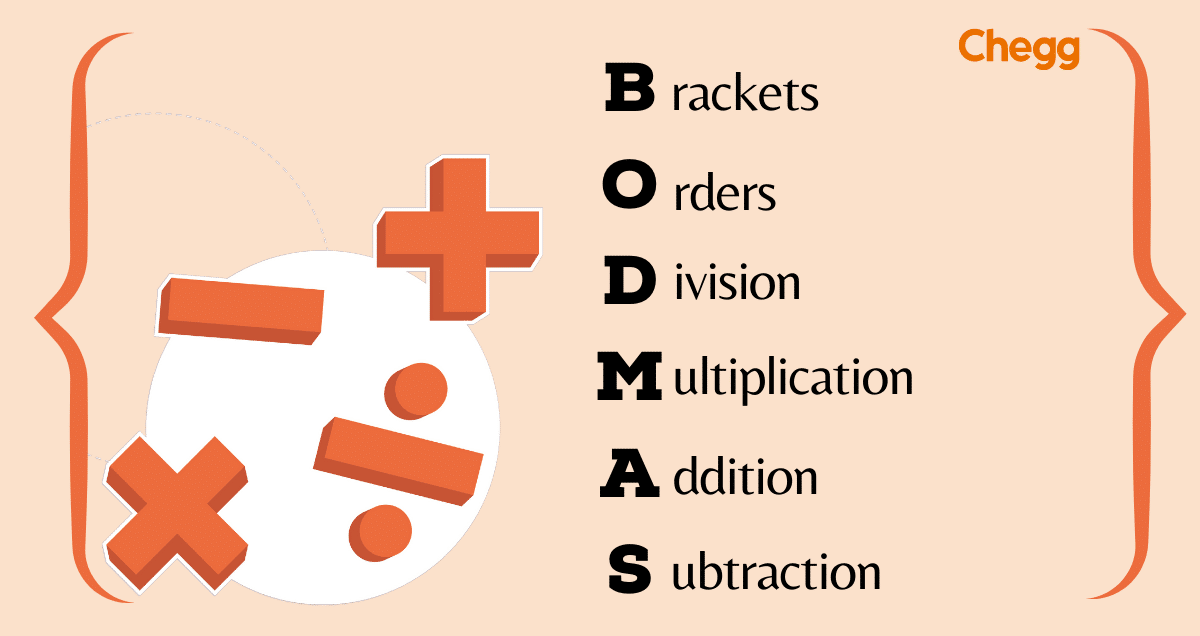
BODMAS Full Form
The BODMAS full form stands for Brackets, Orders, Division, Multiplication, Addition, and Subtraction. BODMAS is used in mathematics to maintain the order of the mathematical operation. Another name of BODMAS is PEDMAS which stands for Parentheses, Exponents, Division, Multiplication, Addition, and Subtraction.
What is BODMAS full form?
A fundamental rule in mathematics is called BODMAS. BODMAS Full form is Brackets, Orders, Division, Multiplication, Addition, and Subtraction, specifies the proper order of operations to do while evaluating mathematical equations. Mathematicians and students can guarantee precise and consistent computations following the BODMAS rule. Look closer at BODMAS’s elements and their role in mathematical operations.
Importance of BODMAS
It’s not always possible to recall the steps by the series when solving a complex summation. The precise series you need to follow to solve the problem, however, will stick in your memory if you can recall the term “BODMAS.” For instance, you will obtain an inaccurate result if you inadvertently multiply a number before dividing.
What does BODMAS stand for?
The BODMAS full form stands for the series of steps that should be taken to simplify or solve mathematical statements. In BODMAS, each letter stands for a particular operation:
- Brackets
Brackets, including parentheses, square brackets, or curly braces, are used to group numbers or expressions. They indicate that the enclosed portion should be evaluated as a single entity before proceeding with the rest of the expression.
- Orders
Orders refer to exponents or powers. In mathematical operations, numbers raised to the power or exponent are given precedence and should be calculated before other operations.
- Division and Multiplication
Division and multiplication are performed from left to right in the order they appear in the expression. These operations are given equal precedence and should be executed before addition and subtraction.
- Addition and Subtraction
Addition and subtraction, like division and multiplication, are performed from left to right. These operations are executed after completing brackets, orders, division, and multiplication.
Breakdown of BODMAS components
Below is the table of BODMAS showing the detailed explanation:
| Components | Explanation |
| Brackets | Calculations can be made simpler using brackets. They aid in determining which components of a statement must be examined first. Mathematicians can reduce complex equations and continue with additional computations by resolving the information contained within the brackets. |
| Orders | Dealing with exponents and powers is a part of orders. Exponents show how often a base number has been multiplied by itself. Mathematicians can effectively manage numbers raised to powers by performing exponentiation operations according to the BODMAS formula. |
| Division and Multiplication | In mathematics, division, and multiplication are basic operations. The BODMAS rule strongly emphasizes carrying out these procedures in the proper sequence, from left to right. Confusion in complex expressions is avoided by following this order, guaranteeing correct and consistent outcomes. |
| Addition and Subtraction | The BODMAS formula’s last operations are addition and subtraction. Mathematicians can conduct addition and subtraction progressively to arrive at the ultimate result once brackets, ordering, division, and multiplication have been resolved. |
Rules and Conditions of BODMAS
Below are some Rules and Conditions for general simplification:
| Condition | Rule |
| x + (y + z) ⇒ x + y + z | In this case, open the bracket first and add the terms. |
| x – (y + z) ⇒ x – y – z | In this case, open the bracket and multiply each term inside it by the negative sign. (All positive terms will be negative and negative terms will be positive) |
| x(y + z) ⇒ xy + xz | Multiply each term enclosed in a bracket by the term outside of it. |
BODMAS Questions with Answers
Let’s look at some step-by-step Brackets, Orders, Division, Multiplication, Addition, and Subtraction (BODMAS full form) rule examples to understand better how it might be used in real-world situations. Consider the following BODMAS question with answers:
- 6 + 3 (8 – 2) 4. Using BODMAS full form, we evaluate the brackets:
- 8 – 2 = 6.
- After that, we divide 6 by 4 to get 1.5.
- Ultimately, we multiply and add 3 x 6 = 18 and 6 x 18 = 24.
- As a consequence, the phrase produces the number 24.
BODMAS full form allows us to efficiently answer complex mathematical equations, providing precise results and consistency between calculations.
Question 1: Solve 7 + 8 ÷ 8 + 9 × 3 − 5
Solution: The problem given is 7 + 8 ÷ 8 + 9 × 3 − 5
The division operation is performed first.
8 ÷ 8 = 1
So, the expression reduces to 7 + 1 + 9 × 3 − 5
The multiplication operation is taken next,
9 × 3 = 27
So, the expression reduces to 7 + 1 + 27 − 5
The Addition operation is
7 + 1 + 27 = 35
The final answer is 35 – 5 = 30.
Question 2: Simplify the expression [22 – 4 (2 + 3)] ÷ 5 + 8
Solution: The problem given is [22 – 4 (2 + 3)] ÷ 5 + 8
The round bracket is (2 + 3) = 5.
The next bracket is 4 (5) = 20.
Take [22 – 20] ÷ 5 + 8
(25 – 16) = 2
The division operation is performed,
2 ÷ 5 = 0.4
Then 0.4 + 8 = 8.4
The final answer is 8.4
BODMAS and Problem-Solving
The BODMAS full form rule extends beyond simplifying expressions. When faced with word problems or mathematical puzzles, applying BODMAS enables us to break down the problem into manageable parts. By identifying the order of operations, we can solve problems systematically and arrive at the correct solutions. Trying BODMAS enhances our mathematical reasoning and problem-solving skills.
Importance of BODMAS in Mathematics
BODMAS is incredibly significant since it removes uncertainty and guarantees accurate results. Adhering to BODMAS full form becomes crucial in equations and higher-level mathematical ideas. It gives mathematicians, physicists, and engineers a standardized method, enabling them to carry out precise calculations and examine intricate mathematical models.
BODMAS in Real-World Applications
BODMAS finds applications in various fields; let us look at them.
- Engineering
Engineers extensively use BODMAS full form in complex structural analysis, electrical circuit design, and fluid dynamics calculations.
- Finance and Accounting
Financial professionals rely on BODMAS to calculate compound interest and investment returns and perform accurate financial modeling.
- Computer Science
BODMAS is vital in programming and algorithm design, where mathematical operations are fundamental.
- Physics
Physicists employ BODMAS when solving energy, motion, and quantum mechanics equations.
For more Full Forms → Click Here
Conclusion
Understanding the BODMAS Full form and the BODMAS formula is essential for accurate and consistent mathematical computations. By considering the components of BODMAS, including brackets, orders, division, multiplication, addition, and subtraction, mathematicians, and students can simplify expressions, solve complex problems, and enhance their problem-solving skills. Try the BODMAS as a fundamental tool in your mathematical journey to ensure precise and reliable results.
| LPG full form | GMT full form |
| LIC full form | SDM full form |
| SUV full form | CPI full form |
| PFMS full form | IMDb full form |
| SC full form | PRO full form |
BODMAS Full Form: FAQs
What is the BODMAS full form?
BODMAS stands for Brackets, Orders, Division, Multiplication, Addition, and Subtraction.
Why is BODMAS important in mathematics?
BODMAS is important as it provides a standardized order of operations, ensuring consistent and accurate calculations.
Can the BODMAS rule be applied to all mathematical expressions?
Yes, the BODMAS rule can be applied to simplify and evaluate all types of mathematical expressions.
Are there any variations or alternatives to the BODMAS rule?
While variations exist in different countries and mathematical curricula, the underlying principles of BODMAS remain the same.
Can I change the order of operations in the BODMAS sums?
No, the order of operations in BODMAS sums should be followed precisely to obtain correct results.
Is BODMAS used universally in all countries?
While the order of operations is widely accepted and followed, the acronym BODMAS may vary across different regions. In some countries, it is referred to as PEMDAS (Parentheses, Exponents, Multiplication, Division, Addition, Subtraction) or other similar variations. However, the underlying principles remain the same.
Who is the father of BODMAS?
Sir Isaac Newton, an English mathematician and physicist from the 17th century, is the father of BODMAS.
Got a question on this topic?